The reason why this side-penetration thing is important was because the last patch is the biggest game-changer to late-game dynamics ever since UKF was released:
- All heavily-armoured vehicles received a hefty rear armour nerf
- SU-85 received a heavy rate-of-fire nerf just so that it can reliably penetrate the frontal armour of heavy tanks.
Since frontal armour remained the same across the board for all heavies, we might soon discover that heavy vehicles are actually a lot squishier when flanked from the side.
Also I'd question your tests work method. How certain can you be that all your tests were done at exact pin point 90 degree angle?
None of them were done at a 90 degree angle.
What I did was pick an angle that seemed narrower than 90 at first, and then I picked an angle that was wider, and it seemed to be wider than 90 degrees.
The degrees of the angles do not matter.
What I care to show is not that at angle X the chances are Y and Z. But if you change your angle slightly:
- The results change
- But the results don't change as dramatically as 90% -> 10%
You can actually see the pictures for yourself in the post I explain the experiment:
https://www.coh2.org/topic/36347/cruzz-s-the-more-you-know/page/43#post_id548065
You can probably determine the angle of the attack by:
- The orientation of the Panther
- The orientation of the gun (which should be aiming at the centre of the Panther)
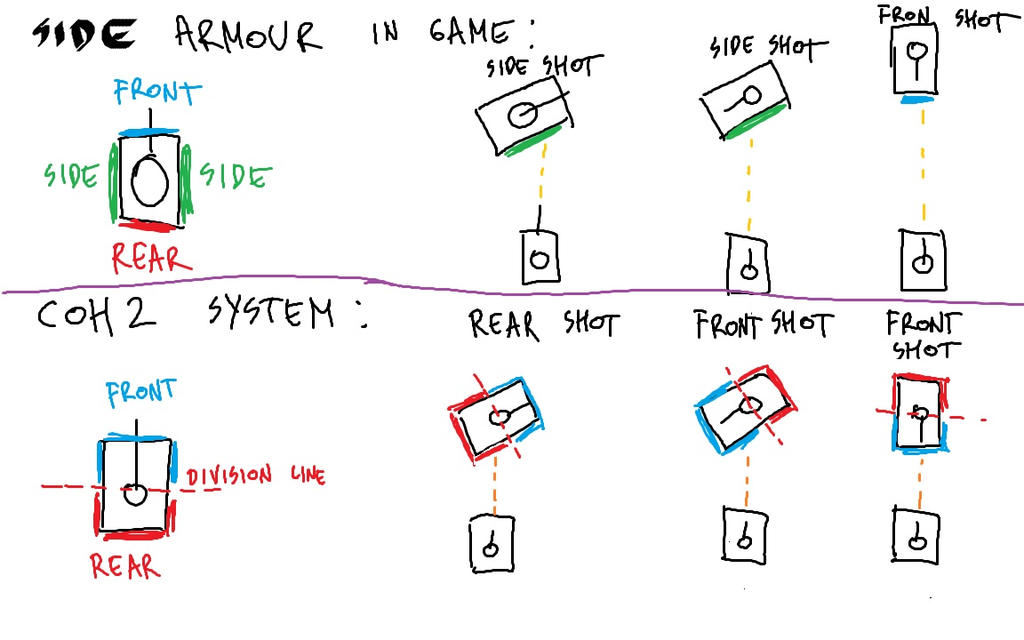
No. It is a side effect of front/ rear armour system exists in CoH2 in which slight angle deviation may result in completely different outcome. If there was side armour in the game each side shot would count as side armour shot but because there is non, you have to possible outcomes dependant on the angle and this itself is an evidence of side armour not being present. I don't know how to put it simpler.
Yes. The value of the effective armour is not fixed. It depends entirely on:
- The angle
- The penetration of the gun
Let's ignore what we have now, for a moment. Suppose CoH2 used a different system:
- You have front and rear armour like now
- Every time a shot lands on the side of the tank, there's a 50% chance rear armour will be used, 50% front armour will be used (regardless of angle)
Now, let's walk through an example.
A Stug has chance to penetration Panther at max range:
- Frontal: 53% (170 / 320)
- Rear: 100% (170 / 110)
You can see that the front armour and rear armour govern the chance of penetration. Now what happens when you attack from the side?
- 50% of the time it hits frontal armour, 50% of the time it hits rear armour
What is the probability that the Stug penetrates when it attacks from the side?
It's equal to the P(rear) * P(penetrate | rear) + P(front) * P (penetrate | front)
P(rear): probability we hit rear armour
P(penetrate | rear): conditional probability to penetrate when we hit rear armour
If we substitute these variables we get:
50% * 100% + 50% * 53% = 76.5%
Thus, the chance to penetrate from the side is equal to 76.5%. This is neither 53% (front) nor 100% (rear). From the perspective of the Stug, with penetration 170, a 76.5% penetration chance is the equivalent to its gun firing at a target with armour 170 / 76.5% = 222.
If we used a gun with different penetration, the value of the effective armour would be different.
Now, in CoH2, the firing angle also seems to affect the value of the effective armour. I don't know exactly how much yet, but it is important to find out how, as this affects engagements.



 Romeo
Romeo
 Mr Smith
Mr Smith
 Luciano
Luciano
 Mr Smith
Mr Smith









